

Thinking in terms of overlapping atomic orbitals is one way for us to explain how chemical bonds form in diatomic molecules. However, to understand how molecules with more than two atoms form stable bonds, we require a more detailed model. As an example, let us consider the water molecule, in which we have one oxygen atom bonding to two hydrogen atoms. Oxygen has the electron configuration 1s 2 2s 2 2p 4 , with two unpaired electrons (one in each of the two 2p orbitals). Valence bond theory would predict that the two [latex]\ce[/latex] bonds form from the overlap of these two 2p orbitals with the 1s orbitals of the hydrogen atoms. If this were the case, the bond angle would be 90°, as shown in Figure 7.5.1 (note that orbitals may sometimes be drawn in an elongated “balloon” shape rather than in a more realistic “plump” shape in order to make the geometry easier to visualize), because p orbitals are perpendicular to each other.. Experimental evidence shows that the bond angle is 104.5°, not 90°. The prediction of the valence bond theory model does not match the real-world observations of a water molecule; a different model is needed.
Quantum-mechanical calculations suggest why the observed bond angles in [latex]\ce[/latex] differ from those predicted by the overlap of the 1s orbital of the hydrogen atoms with the 2p orbitals of the oxygen atom. The mathematical expression known as the wave function, ψ, contains information about each orbital and the wavelike properties of electrons in an isolated atom. When atoms are bound together in a molecule, the wave functions combine to produce new mathematical descriptions that have different shapes. This process of combining the wave functions for atomic orbitals is called hybridization and is mathematically accomplished by the linear combination of atomic orbitals, LCAO, (a technique that we will encounter again later). The new orbitals that result are called hybrid orbital. The valence orbitals in an isolated oxygen atom are a 2s orbital and three 2p orbitals. The valence orbitals in an oxygen atom in a water molecule differ; they consist of four equivalent hybrid orbitals that point approximately toward the corners of a tetrahedron (Figure 7.5.2). Consequently, the overlap of the [latex]\ce[/latex] and [latex]\ce[/latex] orbitals should result in a tetrahedral bond angle (109.5°). The observed angle of 104.5° is experimental evidence for which quantum-mechanical calculations give a useful explanation: Valence bond theory must include a hybridization component to give accurate predictions.

The following ideas are important in understanding hybridization:
In the following sections, we shall discuss the common types of hybrid orbitals.
The beryllium atom in a gaseous [latex]\ce[/latex] molecule is an example of a central atom with no lone pairs of electrons in a linear arrangement of three atoms. There are two regions of valence electron density in the [latex]\ce[/latex] molecule that correspond to the two covalent [latex]\ce[/latex] bonds. To accommodate these two electron domains, two of the Be atom’s four valence orbitals will mix to yield two hybrid orbitals. This hybridization process involves mixing of the valence s orbital with one of the valence p orbitals to yield two equivalent sp hybrid orbital that are oriented in a linear geometry (Figure 7.5.3). In this figure, the set of sp orbitals appears similar in shape to the original p orbital, but there is an important difference. The number of atomic orbitals combined always equals the number of hybrid orbitals formed. The p orbital is one orbital that can hold up to two electrons. The sp set is two equivalent orbitals that point 180° from each other. The two electrons that were originally in the s orbital are now distributed to the two sp orbitals, which are half filled. In gaseous [latex]\ce[/latex], these half-filled hybrid orbitals will overlap with orbitals from the chlorine atoms to form two identical [latex]\sigma[/latex] bonds.

We illustrate the electronic differences in an isolated Be atom and in the bonded Be atom in the orbital energy-level diagram in Figure 7.5.4. These diagrams represent each orbital by a horizontal line (indicating its energy) and each electron by an arrow. Energy increases toward the top of the diagram. We use one upward arrow to indicate one electron in an orbital and two arrows (up and down) to indicate two electrons of opposite spin.

Any central atom surrounded by just two regions of valence electron density in a molecule will exhibit sp hybridization. Other examples include the mercury atom in the linear [latex]\ce[/latex] molecule, the zinc atom in [latex]\ce[/latex], which contains a linear [latex]\ce[/latex] arrangement, and the carbon atoms in [latex]\ce[/latex] and [latex]\ce[/latex].
The valence orbitals of a central atom surrounded by three regions of electron density consist of a set of three sp 2 hybrid orbital and one unhybridized p orbital. This arrangement results from sp 2 hybridization, the mixing of one s orbital and two p orbitals to produce three identical hybrid orbitals oriented in a trigonal planar geometry (Figure 7.5.5).


Although quantum mechanics yields the “plump” orbital lobes as depicted in Figure 7.5.5, sometimes for clarity these orbitals are drawn thinner and without the minor lobes, as in Figure 7.5.6, to avoid obscuring other features of a given illustration.
We will use these “thinner” representations whenever the true view is too crowded to easily visualize.
The observed structure of the borane molecule, [latex]\ce[/latex], suggests sp 2 hybridization for boron in this compound. The molecule is trigonal planar, and the boron atom is involved in three bonds to hydrogen atoms (Figure 7.5.7).

We can illustrate the comparison of orbitals and electron distribution in an isolated boron atom and in the bonded atom in [latex]\ce[/latex] as shown in the orbital energy level diagram in Figure 7.5.8. We redistribute the three valence electrons of the boron atom in the three sp 2 hybrid orbitals, and each boron electron pairs with a hydrogen electron when [latex]\ce[/latex] bonds form.


The valence orbitals of an atom surrounded by a tetrahedral arrangement of bonding pairs and lone pairs consist of a set of four sp 3 hybrid orbital. The hybrids result from the mixing of one s orbital and all three p orbitals that produces four identical sp 3 hybrid orbitals (Figure 7.5.10). Each of these hybrid orbitals points toward a different corner of a tetrahedron.

A molecule of methane, [latex]\ce[/latex], consists of a carbon atom surrounded by four hydrogen atoms at the corners of a tetrahedron. The carbon atom in methane exhibits sp 3 hybridization. We illustrate the orbitals and electron distribution in an isolated carbon atom and in the bonded atom in [latex]\ce[/latex] in Figure 7.5.11. The four valence electrons of the carbon atom are distributed equally in the hybrid orbitals, and each carbon electron pairs with a hydrogen electron when the [latex]\ce[/latex] bonds form.

In a methane molecule, the 1s orbital of each of the four hydrogen atoms overlaps with one of the four sp 3 orbitals of the carbon atom to form a sigma ([latex]\sigma[/latex]) bond. This results in the formation of four strong, equivalent covalent bonds between the carbon atom and each of the hydrogen atoms to produce the methane molecule, [latex]\ce[/latex].
The structure of ethane, [latex]\ce[/latex], is similar to that of methane in that each carbon in ethane has four neighboring atoms arranged at the corners of a tetrahedron—three hydrogen atoms and one carbon atom (Figure 7.5.12). However, in ethane an sp 3 orbital of one carbon atom overlaps end to end with an sp 3 orbital of a second carbon atom to form a σ bond between the two carbon atoms. Each of the remaining sp 3 hybrid orbitals overlaps with an s orbital of a hydrogen atom to form carbon–hydrogen σ bonds. The structure and overall outline of the bonding orbitals of ethane are shown in Figure 7.5.12. The orientation of the two [latex]\ce[/latex] groups is not fixed relative to each other. Experimental evidence shows that rotation around [latex]\sigma[/latex] bonds occurs easily.

An sp 3 hybrid orbital can also hold a lone pair of electrons. For example, the nitrogen atom in ammonia is surrounded by three bonding pairs and a lone pair of electrons directed to the four corners of a tetrahedron. The nitrogen atom is sp 3 hybridized with one hybrid orbital occupied by the lone pair. The molecular structure of water is consistent with a tetrahedral arrangement of two lone pairs and two bonding pairs of electrons. Thus we say that the oxygen atom is sp 3 hybridized, with two of the hybrid orbitals occupied by lone pairs and two by bonding pairs. Since lone pairs occupy more space than bonding pairs, structures that contain lone pairs have bond angles slightly distorted from the ideal. Perfect tetrahedra have angles of 109.5°, but the observed angles in ammonia (107.3°) and water (104.5°) are slightly smaller. Other examples of sp 3 hybridization include [latex]\ce[/latex], [latex]\ce[/latex], and [latex]\ce[/latex].
To describe the five bonding orbitals in a trigonal bipyramidal arrangement, we must use five of the valence shell atomic orbitals (the s orbital, the three p orbitals, and one of the d orbitals), which gives five sp 3 d hybrid orbital. With an octahedral arrangement of six hybrid orbitals, we must use six valence shell atomic orbitals (the s orbital, the three p orbitals, and two of the d orbitals in its valence shell), which gives six sp 3 d 2 hybrid orbital. These hybridizations are only possible for atoms that have d orbitals in their valence subshells (that is, not those in the first or second period).
In a molecule of phosphorus pentachloride, [latex]\ce[/latex], there are five [latex]\ce[/latex] bonds (thus five pairs of valence electrons around the phosphorus atom) directed toward the corners of a trigonal bipyramid. We use the 3s orbital, the three 3p orbitals, and one of the 3d orbitals to form the set of five sp 3 d hybrid orbitals (Figure 7.5.14) that are involved in the P–Cl bonds. Other atoms that exhibit sp 3 d hybridization include the sulfur atom in [latex]\ce[/latex] and the chlorine atoms in [latex]\ce[/latex] and in [latex]\ce[/latex]. (The electrons on fluorine atoms are omitted for clarity.)


The sulfur atom in sulfur hexafluoride, [latex]\ce[/latex], exhibits sp 3 d 2 hybridization. A molecule of sulfur hexafluoride has six bonding pairs of electrons connecting six fluorine atoms to a single sulfur atom (Figure 7.5.15). There are no lone pairs of electrons on the central atom. To bond six fluorine atoms, the 3s orbital, the three 3p orbitals, and two of the 3d orbitals form six equivalent sp 3 d 2 hybrid orbitals, each directed toward a different corner of an octahedron. Other atoms that exhibit sp 3 d 2 hybridization include the phosphorus atom in [latex]>_^,[/latex] the iodine atom in the interhalogens [latex]>_^>[/latex], [latex]\ce_[/latex], [latex]>_^[/latex], [latex]>_^[/latex] and the xenon atom in [latex]\ce_[/latex].

The hybridization of an atom is determined based on the number of regions of electron density that surround it. The geometrical arrangements characteristic of the various sets of hybrid orbitals are shown in Figure 7.5.16. These arrangements are identical to those of the electron-pair geometries predicted by VSEPR theory. VSEPR theory predicts the shapes of molecules, and hybrid orbital theory provides an explanation for how those shapes are formed. To find the hybridization of a central atom, we can use the following guidelines:
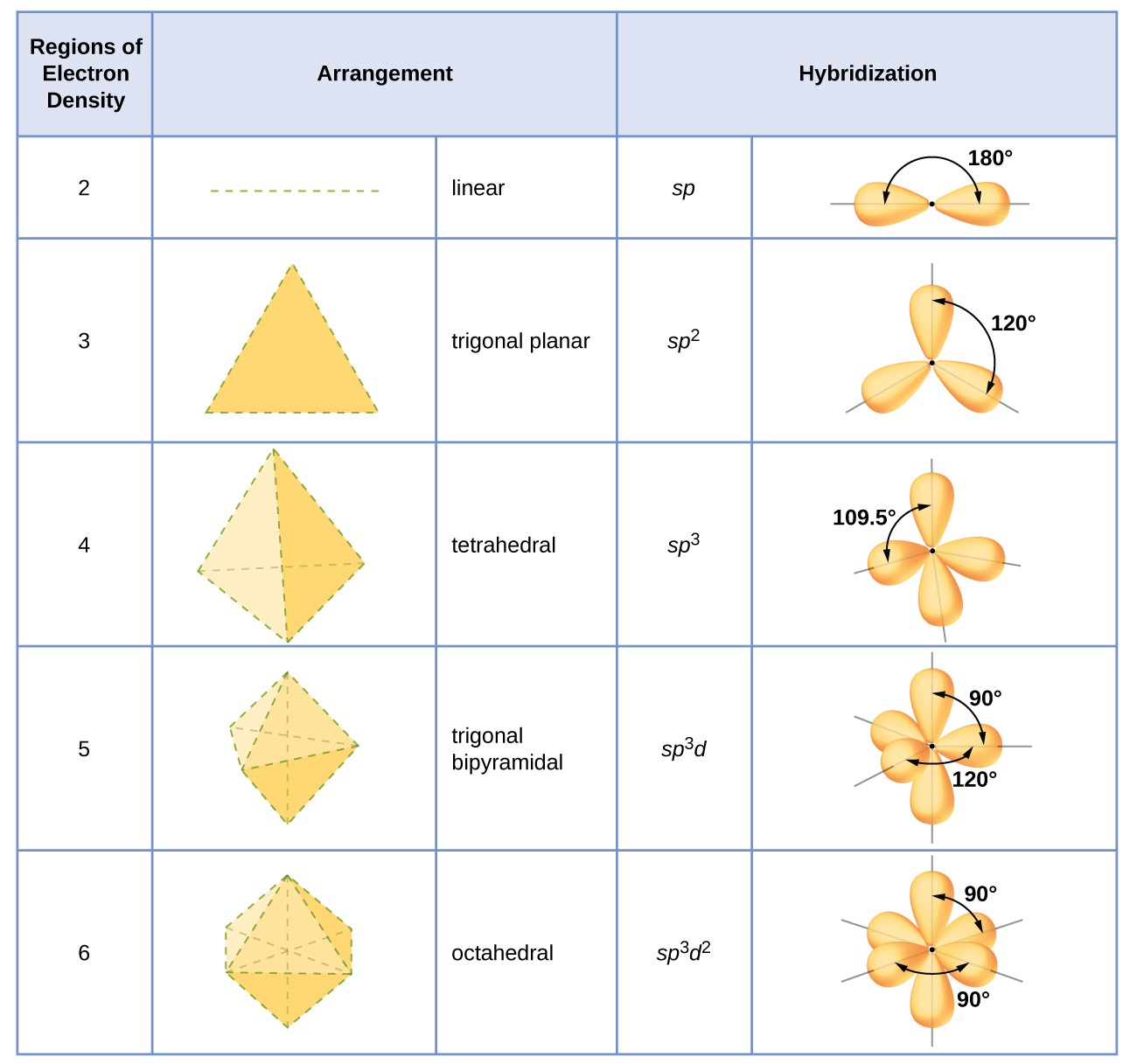
It is important to remember that hybridization was devised to rationalize experimentally observed molecular geometries. The model works well for molecules containing small central atoms, in which the valence electron pairs are close together in space. However, for larger central atoms, the valence-shell electron pairs are farther from the nucleus, and there are fewer repulsions. Their compounds exhibit structures that are often not consistent with VSEPR theory, and hybridized orbitals are not necessary to explain the observed data. For example, we have discussed the [latex]\ce[/latex] bond angle in [latex]\ce[/latex], 104.5°, which is more consistent with sp 3 hybrid orbitals (109.5°) on the central atom than with 2p orbitals (90°). Sulfur is in the same group as oxygen, and H2S has a similar Lewis structure. However, it has a much smaller bond angle (92.1°), which indicates much less hybridization on sulfur than oxygen. Continuing down the group, tellurium is even larger than sulfur, and for [latex]\ce[/latex], the observed bond angle (90°) is consistent with overlap of the 5p orbitals, without invoking hybridization. We invoke hybridization where it is necessary to explain the observed structures.

Ammonium sulfate is important as a fertilizer. What is the hybridization of the sulfur atom in the sulfate ion, [latex]\ce>[/latex]?
The Lewis structure of sulfate shows there are four regions of electron density. The hybridization is sp 3 .

Urea, [latex]\ce[/latex], is sometimes used as a source of nitrogen in fertilizers. What is the hybridization of each nitrogen and carbon atom in urea?
The Lewis structure of urea is

The nitrogen atoms are surrounded by four regions of electron density, which arrange themselves in a tetrahedral electron-pair geometry. The hybridization in a tetrahedral arrangement is sp 3 . This is the hybridization of the nitrogen atoms in urea. The carbon atom is surrounded by three regions of electron density, positioned in a trigonal planar arrangement. The hybridization in a trigonal planar electron pair geometry is sp 2 , which is the hybridization of the carbon atom in urea.
We can use hybrid orbitals, which are mathematical combinations of some or all of the valence atomic orbitals, to describe the electron density around covalently bonded atoms. These hybrid orbitals either form sigma ([latex]\sigma[/latex]) bonds directed toward other atoms of the molecule or contain lone pairs of electrons. We can determine the type of hybridization around a central atom from the geometry of the regions of electron density about it. Two such regions imply sp hybridization; three, sp 2 hybridization; four, sp 3 hybridization; five, sp 3 d hybridization; and six, sp 3 d 2 hybridization. Pi (π) bonds are formed from unhybridized atomic orbitals (p or d orbitals).
2. trigonal planar, sp 2 , trigonal pyramidal (one lone pair on A) sp 3 , T-shaped (two lone pairs on A sp 3 d, or (three lone pair on A) sp 3 d 2
3. Phosphorus and nitrogen can form sp 3 hybrids to form three bonds and hold one lone pair in [latex]\ce[/latex] and [latex]\ce[/latex], respectively. However, nitrogen has no valence d orbitals, so it cannot form a set of sp 3 d hybrid orbitals to bind five fluorine atoms in [latex]\ce[/latex]. Phosphorus has d orbitals and can bind five fluorine atoms with sp 3 d hybrid orbitals in [latex]\ce[/latex].

hybrid orbital: orbital created by combining atomic orbitals on a central atom
hybridization: model that describes the changes in the atomic orbitals of an atom when it forms a covalent compound
sp hybrid orbital: one of a set of two orbitals with a linear arrangement that results from combining one s and one p orbital
sp 2 hybrid orbital: one of a set of three orbitals with a trigonal planar arrangement that results from combining one s and two p orbitals
sp 3 hybrid orbital: one of a set of four orbitals with a tetrahedral arrangement that results from combining one s and three p orbitals
sp 3 d hybrid orbital: one of a set of five orbitals with a trigonal bipyramidal arrangement that results from combining one s, three p, and one d orbital
sp 3 d 2 hybrid orbital: one of a set of six orbitals with an octahedral arrangement that results from combining one s, three p, and two d orbitals
Licenses and Attributions (Click to expand)CC licensed content, Shared previously
one of a set of two orbitals with a linear arrangement that results from combining one s and one p orbital
× Close definitionorbital created by combining atomic orbitals on a central atom
× Close definitionone of a set of two orbitals with a linear arrangement that results from combining one s and one p orbital
× Close definitionone of a set of three orbitals with a trigonal planar arrangement that results from combining one s and two p orbitals
× Close definitionone of a set of four orbitals with a tetrahedral arrangement that results from combining one s and three p orbitals
× Close definitionone of a set of five orbitals with a trigonal bipyramidal arrangement that results from combining one s, three p, and one d orbital
× Close definitionone of a set of six orbitals with an octahedral arrangement that results from combining one s, three p, and two d orbitals
× Close definitionChemistry Fundamentals Copyright © by Dr. Julie Donnelly, Dr. Nicole Lapeyrouse, and Dr. Matthew Rex is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.